Examples of solving logarithmic equations with roots. Solving Logarithmic Equations - Final Lesson

Many students get stuck on equations of this kind. At the same time, the tasks themselves are by no means complex - it is enough just to perform a competent variable substitution, for which you should learn how to isolate stable expressions.

In addition to this lesson, you will find a rather voluminous independent work, consisting of two options with 6 tasks each.

Grouping method

Today we will analyze two logarithmic equations, one of which cannot be solved "throughout" and requires special transformations, and the second ... however, I will not tell everything at once. Watch the video, download independent work - and learn how to solve complex problems.

So, grouping and taking the common factors out of the bracket. In addition, I will tell you what pitfalls the domain of definition of logarithms carries, and how small remarks on the domain of definitions can significantly change both the roots and the whole solution.

Let's start with the grouping. We need to solve the following logarithmic equation:

log 2 x log 2 (x − 3) + 1 = log 2 (x 2 − 3x )

First of all, we note that x 2 − 3x can be factorized:

log 2 x (x − 3)

Then we remember the wonderful formula:

log a fg = log a f + log a g

Immediately a small note: this formula works fine when a, f and g are ordinary numbers. But when there are functions instead of them, these expressions cease to be equal in rights. Imagine this hypothetical situation:

f< 0; g < 0

In this case, the product fg will be positive, therefore, log a ( fg ) will exist, but log a f and log a g will not exist separately, and we cannot perform such a transformation.

Ignoring this fact will lead to a narrowing of the domain of definition and, as a result, to the loss of roots. Therefore, before performing such a transformation, it is necessary to make sure in advance that the functions f and g are positive.

In our case, everything is simple. Since there is a function log 2 x in the original equation, then x > 0 (after all, the variable x is in the argument). There is also log 2 (x − 3), so x − 3 > 0.

Therefore, in the function log 2 x (x − 3) each factor will be greater than zero. Therefore, we can safely decompose the product into the sum:

log 2 x log 2 (x − 3) + 1 = log 2 x + log 2 (x − 3)

log 2 x log 2 (x − 3) + 1 − log 2 x − log 2 (x − 3) = 0

At first glance, it may seem that it has not become easier. On the contrary: the number of terms only increased! To understand how to proceed further, we introduce new variables:

log 2 x = a

log 2 (x − 3) = b

a b + 1 − a − b = 0

And now we group the third term with the first:

(a b - a) + (1 - b) = 0

a (1 b - 1) + (1 - b ) = 0

Note that both the first and second brackets contain b − 1 (in the second case, you will have to take the “minus” out of the bracket). Let's factorize our construction:

a (1 b − 1) − (b − 1) = 0

(b − 1)(a 1 − 1) = 0

And now we recall our wonderful rule: the product is equal to zero when at least one of the factors is equal to zero:

b − 1 = 0 ⇒ b = 1;

a − 1 = 0 ⇒ a = 1.

Let's remember what b and a are. We get two simple logarithmic equations in which all that remains is to get rid of the signs of log and equate the arguments:

log 2 x = 1 ⇒ log 2 x = log 2 2 ⇒ x 1 =2;

log 2 (x − 3) = 1 ⇒ log 2 (x − 3) = log 2 2 ⇒ x 2 = 5

We got two roots, but this is not a solution to the original logarithmic equation, but only candidates for the answer. Now let's check the domain. For the first argument:

x > 0

Both roots satisfy the first requirement. Let's move on to the second argument:

x − 3 > 0 ⇒ x > 3

But here already x = 2 does not satisfy us, but x = 5 suits us quite well. Therefore, the only answer is x = 5.

We pass to the second logarithmic equation. At first glance, it is much simpler. However, in the process of solving it, we will consider subtle points related to the domain of definition, ignorance of which significantly complicates the life of novice students.

log 0.7 (x 2 - 6x + 2) = log 0.7 (7 - 2x)

Before us is the canonical form of the logarithmic equation. You don't need to convert anything - even the bases are the same. Therefore, we simply equate the arguments:

x 2 - 6x + 2 = 7 - 2x

x 2 - 6x + 2 - 7 + 2x = 0

x 2 - 4x - 5 = 0

Before us is the given quadratic equation, it is easily solved using the Vieta formulas:

(x − 5) (x + 1) = 0;

x − 5 = 0 ⇒ x = 5;

x + 1 = 0 ⇒ x = −1.

But these roots are not definitive answers yet. It is necessary to find the domain of definition, since there are two logarithms in the original equation, i.e. it is strictly necessary to take into account the domain of definition.

So, let's write out the domain of definition. On the one hand, the argument of the first logarithm must be greater than zero:

x 2 − 6x + 2 > 0

On the other hand, the second argument must also be greater than zero:

7 − 2x > 0

These requirements must be met at the same time. And here the most interesting begins. Of course, we can solve each of these inequalities, then intersect them and find the domain of the entire equation. But why make life so difficult for yourself?

Let's notice one subtlety. Getting rid of log signs, we equate arguments. This implies that the requirements x 2 − 6x + 2 > 0 and 7 − 2x > 0 are equivalent. As a consequence, either of the two inequalities can be crossed out. Let's cross out the most difficult, and leave the usual linear inequality for ourselves:

-2x > -7

x< 3,5

Since we were dividing both sides by a negative number, the sign of the inequality has changed.

So, we have found the ODZ without any square inequalities, discriminants and intersections. Now it remains just to choose the roots that lie on this interval. Obviously, only x = −1 will suit us, because x = 5 > 3.5.

You can write down the answer: x = 1 is the only solution to the original logarithmic equation.

The conclusions from this logarithmic equation are as follows:

1. Don't be afraid to factor logarithms, and then factor the sum of logarithms. However, remember that by breaking the product into the sum of two logarithms, you thereby narrow the domain of definition. Therefore, before performing such a conversion, be sure to check what the scope requirements are. Most often, no problems arise, but it does not hurt to play it safe once again.
2. When getting rid of the canonical form, try to optimize the calculations. In particular, if we are required that f > 0 and g > 0, but in the equation itself f = g , then we boldly cross out one of the inequalities, leaving only the simplest one for ourselves. In this case, the domain of definition and answers will not suffer in any way, but the amount of calculations will be significantly reduced.

That, in fact, is all that I wanted to tell about the grouping. :)

Typical mistakes in solving

Today we will analyze two typical logarithmic equations that many students stumble over. On the example of these equations, we will see what mistakes are most often made in the process of solving and transforming the original expressions.

Fractional-rational equations with logarithms

It should be noted right away that this is a rather insidious type of equation, in which a fraction with a logarithm somewhere in the denominator is not always immediately present. However, in the process of transformations, such a fraction will necessarily arise.

At the same time, be careful: in the process of transformations, the initial domain of definition of logarithms can change significantly!

We turn to even more rigid logarithmic equations containing fractions and variable bases. In order to do more in one short lesson, I will not tell an elementary theory. Let's go straight to the tasks:

4 log 25 (x − 1) − log 3 27 + 2 log x − 1 5 = 1

Looking at this equation, someone will ask: “What does the fractional rational equation have to do with it? Where is the fraction in this equation? Let's not rush and take a closer look at each term.

First term: 4 log 25 (x − 1). The base of the logarithm is a number, but the argument is a function of x . We can't do anything about this yet. Move on.

The next term is log 3 27. Recall that 27 = 3 3 . Therefore, we can rewrite the entire logarithm as follows:

log 3 27 = 3 3 = 3

So the second term is just a three. The third term: 2 log x − 1 5. Not everything is simple here either: the base is a function, the argument is an ordinary number. I propose to flip the whole logarithm according to the following formula:

log a b = 1/log b a

Such a transformation can only be performed if b ≠ 1. Otherwise, the logarithm that will be obtained in the denominator of the second fraction simply will not exist. In our case, b = 5, so everything is fine:

2 log x − 1 5 = 2/log 5 (x − 1)

Let's rewrite the original equation taking into account the obtained transformations:

4 log 25 (x − 1) − 3 + 2/ log 5 (x − 1) = 1

We have log 5 (x − 1) in the denominator of the fraction, and log 25 (x − 1) in the first term. But 25 \u003d 5 2, so we take out the square from the base of the logarithm according to the rule:

In other words, the exponent at the base of the logarithm becomes the fraction at the front. And the expression will be rewritten like this:

4 1/2 log 5 (x − 1) − 3 + 2/ log 5 (x − 1) − 1 = 0

We ended up with a long equation with a bunch of identical logarithms. Let's introduce a new variable:

log 5 (x − 1) = t;

2t − 4 + 2/t = 0;

But this is already a fractional-rational equation, which is solved by means of algebra of grades 8-9. First, let's break it down into two:

t − 2 + 1/t = 0;

(t 2 − 2t + 1)/t = 0

The exact square is in brackets. Let's roll it up:

(t − 1) 2 /t = 0

A fraction is zero when its numerator is zero and its denominator is non-zero. Never forget this fact:

(t − 1) 2 = 0

t=1

t ≠ 0

Let's remember what t is:

log 5 (x − 1) = 1

log 5 (x − 1) = log 5 5

We get rid of the log signs, equate their arguments, and we get:

x − 1 = 5 ⇒ x = 6

Everybody. Problem solved. But let's go back to the original equation and remember that there were two logarithms with the x variable at once. Therefore, you need to write out the domain of definition. Since x − 1 is in the logarithm argument, this expression must be greater than zero:

x − 1 > 0

On the other hand, the same x − 1 is also present in the base, so it must differ from one:

x − 1 ≠ 1

Hence we conclude:

x > 1; x ≠ 2

These requirements must be met at the same time. The value x = 6 satisfies both requirements, so x = 6 is the final solution to the logarithmic equation.

Let's move on to the second task:

Again, let's not rush and look at each term:

log 4 (x + 1) - there is a four at the base. The usual number, and you can not touch it. But last time we stumbled upon an exact square at the base, which had to be taken out from under the sign of the logarithm. Let's do the same now:

log 4 (x + 1) = 1/2 log 2 (x + 1)

The trick is that we already have a logarithm with variable x , albeit in the base - it is the inverse of the logarithm that we just found:

8 log x + 1 2 = 8 (1/log 2 (x + 1)) = 8/log 2 (x + 1)

The next term is log 2 8. This is a constant, since both the argument and the base are ordinary numbers. Let's find the value:

log 2 8 = log 2 2 3 = 3

We can do the same with the last logarithm:

Now let's rewrite the original equation:

1/2 log 2 (x + 1) + 8/log 2 (x + 1) − 3 − 1 = 0;

log 2 (x + 1)/2 + 8/log 2 (x + 1) − 4 = 0

Let's bring everything to a common denominator:

Before us is again a fractional-rational equation. Let's introduce a new variable:

t = log 2 (x + 1)

Let's rewrite the equation taking into account the new variable:

Be careful: at this step, I swapped the terms. The numerator of the fraction is the square of the difference:

Like last time, a fraction is zero when its numerator is zero and its denominator is non-zero:

(t − 4) 2 = 0 ⇒ t = 4;

t ≠ 0

We got one root that satisfies all the requirements, so we return to the x variable:

log 2 (x + 1) = 4;

log 2 (x + 1) = log 2 2 4;

x + 1 = 16;

x=15

That's it, we've solved the equation. But since there were several logarithms in the original equation, it is necessary to write out the domain of definition.

So, the expression x + 1 is in the argument of the logarithm. Therefore, x + 1 > 0. On the other hand, x + 1 is also present in the base, i.e. x + 1 ≠ 1. Total:

0 ≠ x > −1

Does the found root satisfy these requirements? Undoubtedly. Therefore, x = 15 is the solution to the original logarithmic equation.

Finally, I would like to say the following: if you look at the equation and understand that you have to solve something complex and non-standard, try to highlight stable structures, which will later be denoted by another variable. If some terms do not contain the variable x at all, they can often be simply calculated.

That's all I wanted to talk about today. I hope this lesson will help you in solving complex logarithmic equations. Watch other video tutorials, download and solve independent work, and see you in the next video!

Algebra Grade 11

Topic: "Methods for solving logarithmic equations"

Lesson Objectives:

educational: the formation of knowledge about different ways of solving logarithmic equations, the ability to apply them in each specific situation and choose any method for solving;

developing: development of skills to observe, compare, apply knowledge in a new situation, identify patterns, generalize; formation of skills of mutual control and self-control;

educational: education of a responsible attitude to educational work, careful perception of the material in the lesson, accuracy of record keeping.

Lesson type: a lesson of familiarization with new material.

"The invention of logarithms, by shortening the work of the astronomer, has lengthened his life."
French mathematician and astronomer P.S. Laplace

During the classes

I. Setting the goal of the lesson

The studied definition of the logarithm, the properties of logarithms and the logarithmic function will allow us to solve logarithmic equations. All logarithmic equations, no matter how complex they are, are solved using the same algorithms. We will consider these algorithms today in the lesson. There are few of them. If you master them, then any equation with logarithms will be feasible for each of you.

Write in your notebook the topic of the lesson: "Methods for solving logarithmic equations." I invite everyone to cooperation.

II. Updating of basic knowledge

Let's get ready to study the topic of the lesson. You solve each task and write down the answer, you can not write the condition. Work in pairs.

1) For what values ​​of x does the function make sense:

(Answers are checked for each slide and errors are sorted out)

2) Do the function graphs match?

3) Rewrite the equalities as logarithmic equalities:

4) Write the numbers as logarithms with base 2:

5) Calculate:

6) Try to restore or complete the missing elements in these equalities.

III. Introduction to new material

The statement is shown on the screen:

"The equation is the golden key that unlocks all mathematical sesame."
Modern Polish mathematician S. Koval

Try to formulate the definition of a logarithmic equation. (An equation containing the unknown under the sign of the logarithm).

Consider the simplest logarithmic equation:logax = b(where a>0, a ≠ 1). Since the logarithmic function increases (or decreases) on the set of positive numbers and takes all real values, it follows from the root theorem that for any b, this equation has, and moreover, only one solution, and a positive one.

Remember the definition of a logarithm. (The logarithm of the number x to the base a is the exponent to which the base a must be raised to get the number x). It immediately follows from the definition of the logarithm that ain is such a solution.

Write down the title: Methods for solving logarithmic equations

1. By definition of the logarithm.

This is how simple equations of the form are solved.

Consider No. 514(a): Solve the equation

How do you propose to solve it? (By definition of logarithm)

Decision. , Hence 2x - 4 = 4; x = 4.

In this task, 2x - 4 > 0, since > 0, therefore, extraneous roots cannot appear, and there is no need to check. The condition 2x - 4 > 0 is not necessary to write out in this task.

2. Potentiation(transition from the logarithm of the given expression to this expression itself).

Consider No. 519(g): log5(x2+8)-log5(x+1)=3log5 2

What feature did you notice? (The bases are the same and the logarithms of the two expressions are equal). What can be done? (potentiate).

In this case, it should be taken into account that any solution is contained among all x for which the logarithm expressions are positive.

Solution: ODZ:

X2+8>0 extra inequality

log5(x2+8) = log5 23+ log5(x+1)

log5(x2+8)=log5(8x+8)

Potentiate the original equation

we get the equation x2+8= 8x+8

We solve it: x2-8x=0

Answer: 0; eight

In general transition to an equivalent system:

The equation

(The system contains a redundant condition - one of the inequalities can be ignored).

Question to the class: Which of these three solutions did you like the most? (Discussion of methods).

You have the right to decide in any way.

3. Introduction of a new variable.

Consider No. 520(g). .

What did you notice? (This is a quadratic equation for log3x) Any suggestions? (Introduce new variable)

Decision. ODZ: x > 0.

Let , then the equation will take the form:. Discriminant D > 0. Roots by Vieta's theorem:.

Let's return to the replacement: or .

Solving the simplest logarithmic equations, we get:

Answer: 27;

4. Logarithm of both sides of the equation.

Solve the equation:.

Solution: ODZ: x>0, take the logarithm of both sides of the equation in base 10:

Apply the property of the logarithm of the degree:

(lgx + 3) lgx = 4

Let lgx = y, then (y + 3)y = 4

, (D > 0) the roots according to the Vieta theorem: y1 = -4 and y2 = 1.

Let's return to the replacement, we get: lgx = -4,; logx = 1, .

Answer: 0.0001; 10.

5. Reduction to one base.

No. 523(c). Solve the equation:

Solution: ODZ: x>0. Let's move on to base 3.

6. Functional-graphical method.

№ 509(d). Solve graphically the equation: = 3 - x.

How do you propose to solve? (Construct graphs of two functions y \u003d log2x and y \u003d 3 - x by points and look for the abscissa of the intersection points of the graphs).

See your solution on the slide.

Is there a way to avoid plotting . It is as follows : if one of the functions y = f(x) increases and the other y = g(x) decreases on the interval X, then the equation f(x)=g(x) has at most one root on the interval X.

If there is a root, then it can be guessed.

In our case, the function increases for x>0, and the function y \u003d 3 - x decreases for all values ​​of x, including x>0, which means that the equation has no more than one root. Note that for x = 2, the equation turns into a true equality, since .

“The correct application of methods can be learned,
only by applying them to various examples.
Danish historian of mathematics G. G. Zeiten

IV. Homework

P. 39 consider example 3, solve No. 514 (b), No. 529 (b), No. 520 (b), No. 523 (b)

V. Summing up the lesson

What methods for solving logarithmic equations did we consider in the lesson?

In the next lessons, we will look at more complex equations. To solve them, the studied methods are useful.

Showing the last slide:

“What is more than anything in the world?
Space.
What is the wisest?
Time.
What is the most enjoyable?
Achieve what you want."
Thales

I want everyone to achieve what they want. Thank you for your cooperation and understanding.

As you know, when multiplying expressions with powers, their exponents always add up (a b * a c = a b + c). This mathematical law was derived by Archimedes, and later, in the 8th century, the mathematician Virasen created a table of integer indicators. It was they who served for the further discovery of logarithms. Examples of using this function can be found almost everywhere where it is required to simplify cumbersome multiplication to simple addition. If you spend 10 minutes reading this article, we will explain to you what logarithms are and how to work with them. Simple and accessible language.

Definition in mathematics

The logarithm is an expression of the following form: log a b=c, that is, the logarithm of any non-negative number (that is, any positive) "b" according to its base "a" is considered the power of "c", to which it is necessary to raise the base "a", so that in the end get the value "b". Let's analyze the logarithm using examples, let's say there is an expression log 2 8. How to find the answer? It's very simple, you need to find such a degree that from 2 to the required degree you get 8. Having done some calculations in your mind, we get the number 3! And rightly so, because 2 to the power of 3 gives the number 8 in the answer.

Varieties of logarithms

For many pupils and students, this topic seems complicated and incomprehensible, but in fact, logarithms are not so scary, the main thing is to understand their general meaning and remember their properties and some rules. There are three distinct kinds of logarithmic expressions:

1. Natural logarithm ln a, where the base is the Euler number (e = 2.7).
2. Decimal a, where the base is 10.
3. The logarithm of any number b to the base a>1.

Each of them is solved in a standard way, including simplification, reduction and subsequent reduction to one logarithm using logarithmic theorems. To obtain the correct values ​​​​of logarithms, one should remember their properties and the order of actions in their decisions.

Rules and some restrictions

In mathematics, there are several rules-limitations that are accepted as an axiom, that is, they are not subject to discussion and are true. For example, it is impossible to divide numbers by zero, and it is also impossible to extract the root of an even degree from negative numbers. Logarithms also have their own rules, following which you can easily learn how to work even with long and capacious logarithmic expressions:

• the base "a" must always be greater than zero, and at the same time not be equal to 1, otherwise the expression will lose its meaning, because "1" and "0" to any degree are always equal to their values;
• if a > 0, then a b > 0, it turns out that "c" must be greater than zero.

How to solve logarithms?

For example, the task was given to find the answer to the equation 10 x \u003d 100. It is very easy, you need to choose such a power, raising the number ten to which we get 100. This, of course, is 10 2 \u003d 100.

Now let's represent this expression as a logarithmic one. We get log 10 100 = 2. When solving logarithms, all actions practically converge to finding the degree to which the base of the logarithm must be entered in order to obtain a given number.

To accurately determine the value of an unknown degree, you must learn how to work with a table of degrees. It looks like this:

As you can see, some exponents can be guessed intuitively if you have a technical mindset and knowledge of the multiplication table. However, larger values ​​will require a power table. It can be used even by those who do not understand anything at all in complex mathematical topics. The left column contains numbers (base a), the top row of numbers is the value of the power c, to which the number a is raised. At the intersection in the cells, the values ​​of the numbers are determined, which are the answer (a c =b). Let's take, for example, the very first cell with the number 10 and square it, we get the value 100, which is indicated at the intersection of our two cells. Everything is so simple and easy that even the most real humanist will understand!

Equations and inequalities

It turns out that under certain conditions, the exponent is the logarithm. Therefore, any mathematical numerical expressions can be written as a logarithmic equation. For example, 3 4 =81 can be written as the logarithm of 81 to base 3, which is four (log 3 81 = 4). For negative powers, the rules are the same: 2 -5 = 1/32 we write as a logarithm, we get log 2 (1/32) = -5. One of the most fascinating sections of mathematics is the topic of "logarithms". We will consider examples and solutions of equations a little lower, immediately after studying their properties. Now let's look at what inequalities look like and how to distinguish them from equations.

An expression of the following form is given: log 2 (x-1) > 3 - it is a logarithmic inequality, since the unknown value "x" is under the sign of the logarithm. And also in the expression two quantities are compared: the logarithm of the desired number in base two is greater than the number three.

The most important difference between logarithmic equations and inequalities is that equations with logarithms (for example, the logarithm of 2 x = √9) imply one or more specific numerical values ​​in the answer, while when solving the inequality, both the range of acceptable values ​​and the points breaking this function. As a consequence, the answer is not a simple set of individual numbers, as in the answer of the equation, but a continuous series or set of numbers.

Basic theorems about logarithms

When solving primitive tasks on finding the values ​​of the logarithm, its properties may not be known. However, when it comes to logarithmic equations or inequalities, first of all, it is necessary to clearly understand and apply in practice all the basic properties of logarithms. We will get acquainted with examples of equations later, let's first analyze each property in more detail.

1. The basic identity looks like this: a logaB =B. It only applies if a is greater than 0, not equal to one, and B is greater than zero.
2. The logarithm of the product can be represented in the following formula: log d (s 1 * s 2) = log d s 1 + log d s 2. In this case, the prerequisite is: d, s 1 and s 2 > 0; a≠1. You can give a proof for this formula of logarithms, with examples and a solution. Let log a s 1 = f 1 and log a s 2 = f 2 , then a f1 = s 1 , a f2 = s 2. We get that s 1 *s 2 = a f1 *a f2 = a f1+f2 (degree properties ), and further by definition: log a (s 1 *s 2)= f 1 + f 2 = log a s1 + log a s 2, which was to be proved.
3. The logarithm of the quotient looks like this: log a (s 1 / s 2) = log a s 1 - log a s 2.
4. The theorem in the form of a formula takes the following form: log a q b n = n/q log a b.

This formula is called "property of the degree of the logarithm". It resembles the properties of ordinary degrees, and it is not surprising, because all mathematics rests on regular postulates. Let's look at the proof.

Let log a b \u003d t, it turns out a t \u003d b. If you raise both parts to the power m: a tn = b n ;

but since a tn = (a q) nt/q = b n , hence log a q b n = (n*t)/t, then log a q b n = n/q log a b. The theorem has been proven.

Examples of problems and inequalities

The most common types of logarithm problems are examples of equations and inequalities. They are found in almost all problem books, and are also included in the mandatory part of exams in mathematics. To enter a university or pass entrance tests in mathematics, you need to know how to solve such tasks correctly.

Unfortunately, there is no single plan or scheme for solving and determining the unknown value of the logarithm, however, certain rules can be applied to each mathematical inequality or logarithmic equation. First of all, you should find out whether the expression can be simplified or reduced to a general form. You can simplify long logarithmic expressions if you use their properties correctly. Let's get to know them soon.

When solving logarithmic equations, it is necessary to determine what kind of logarithm we have before us: an example of an expression may contain a natural logarithm or a decimal one.

Here are examples ln100, ln1026. Their solution boils down to the fact that you need to determine the degree to which the base 10 will be equal to 100 and 1026, respectively. For solutions of natural logarithms, one must apply logarithmic identities or their properties. Let's look at examples of solving logarithmic problems of various types.

How to Use Logarithm Formulas: With Examples and Solutions

So, let's look at examples of using the main theorems on logarithms.

1. The property of the logarithm of the product can be used in tasks where it is necessary to decompose a large value of the number b into simpler factors. For example, log 2 4 + log 2 128 = log 2 (4*128) = log 2 512. The answer is 9.
2. log 4 8 = log 2 2 2 3 = 3/2 log 2 2 = 1.5 - as you can see, using the fourth property of the degree of the logarithm, we managed to solve at first glance a complex and unsolvable expression. It is only necessary to factorize the base and then take the exponent values ​​out of the sign of the logarithm.

Tasks from the exam

Logarithms are often found in entrance exams, especially a lot of logarithmic problems in the Unified State Exam (state exam for all school graduates). Usually these tasks are present not only in part A (the easiest test part of the exam), but also in part C (the most difficult and voluminous tasks). The exam implies an accurate and perfect knowledge of the topic "Natural logarithms".

Examples and problem solving are taken from the official versions of the exam. Let's see how such tasks are solved.

Given log 2 (2x-1) = 4. Solution:
let's rewrite the expression, simplifying it a little log 2 (2x-1) = 2 2 , by the definition of the logarithm we get that 2x-1 = 2 4 , therefore 2x = 17; x = 8.5.

• All logarithms are best reduced to the same base so that the solution is not cumbersome and confusing.
• All expressions under the sign of the logarithm are indicated as positive, therefore, when taking out the exponent of the exponent of the expression, which is under the sign of the logarithm and as its base, the expression remaining under the logarithm must be positive.

The purpose of the lesson: repeat students' knowledge about the logarithm of a number, its properties; learn how to solve logarithmic equations and consolidate them when doing exercises.

Tasks:

Educational: repeat the definition and basic properties of logarithms, be able to apply them in calculating logarithms, in solving logarithmic equations;

Developing: to form the ability to solve logarithmic equations;

Educational: to cultivate perseverance, independence; instill interest in the subject

Lesson type: lesson learning new material.

Required technical equipment:computer, projector, screen.

Structure and course of the lesson:

1. Organizing time.

Teacher .

Hello, have a seat! Today the topic of our lesson is "Solution of logarithmic equations", in which we will get acquainted with the ways to solve them using the definition and properties of logarithms.(slide number 1)

1. oral work.

Consolidation of the concept of the logarithm, repetition of its basic properties and properties of the logarithmic function:

1. Theory warm-up:

1. Define the logarithm.(slide number 2)

2. Is it possible to find the logarithm of any number?

3. What number can be at the base of the logarithm?

4. Function y=log 0.8 x is increasing or decreasing? Why?

5. What values ​​can a logarithmic function take?

6. What logarithms are called decimal, natural?

7. What are the main properties of logarithms.(slide number 3)

8. Is it possible to move from one base of the logarithm to another? How to do it?(slide number 4)

2. Work on the card (3-4 students):

Card number 1: Calculate: a) log 6 4 + log 6 9 =

B) log 1/3 36 - log 1/3 12 =

Solve equation: log 5 x \u003d 4 log 5 3 - 1/3 log 5 27

Card #2:

Calculate: a) log211 - log244 =

B) log1/64 + log1/69 =

Solve equation: log 7 x \u003d 2 log 7 5 + 1/2 log 7 36 - 1/3 log 7 125.

Frontal class survey (oral exercises)

Calculate: (slide number 5)

Compare numbers: (slide number 6)

1. log ½ e and log ½ π;
2. log 2 √5/2 and log 2 √3/2.

Find out the sign of an expression log 0.8 3 log 6 2/3. (slide number 7)

1. Checking homework:

The following exercises were assigned to the house: No. 327 (non-hour), 331 (non-hour), 333 (2) and 390 (6). Check the answers to these tasks and answer the students' questions.

1. Learning new material:

Definition: An equation containing a variable under the sign of the logarithm is called a logarithmic equation.

The simplest example of a logarithmic equation is the equation
log a x \u003d c (a\u003e 0, a ≠ 1)
Ways to solve logarithmic equations:(slide number 8)

1. Solution of equations based on the definition of the logarithm.(slide number 9)

log a x = c (a > 0, a≠ 1) has the solution x = a with .

Based on the definition of the logarithm, equations are solved in which:

• given the bases and the number, the logarithm is determined,
• Given the logarithm and base, a number is determined
• the base is determined by the given number and the logarithm.

Examples:

log 2 128= x, log 16 x = ¾, log x 27= 3,

2 x \u003d 128, x \u003d 16 ¾, x 3 \u003d 27,

2 x \u003d 2 7, x \u003d 2 3, x 3 \u003d 3 3,

x \u003d 7. x = 8. x = 3.

a) log 7 (3x-1)=2 (answer: x=3 1/3)

b) log 2 (7-8x)=2 (answer: x=3/8).

1. potentiation method.(slide number 10)

By potentiation is meant the transition from an equality containing logarithms to an equality that does not contain them, i.e.

Log a f(x) = log a g(x), then f(x) = g(x), provided that f(x)>0, g(x)>0, a>0, a≠ 1.

Example:

Solve the Equation =

ODZ:

3x-1>0; x>1/3

6x+8>0.

3x-1=6x+8

3x=9

x=-3

3 >1/3 - incorrect

Answer: There are no solutions.

lg(x 2 -2) \u003d lg x (answer: x \u003d 2)

1. Equations solved by applying the basic logarithmic identity.(slide number 11)

Example:

Solve the Equation=log 2 (6-x)

ODZ:

6-x>0;

x>0;

x≠1;

log 2 x 2 >0;

x 2 >0.

System solution: (0;1)Ụ (1;6).

Log 2 (6-x)

x 2 = 6 x

x 2 + x-6 = 0

x=-3 does not belong to ODZ.

x=2 belongs to the ODZ.

Answer: x=2

With class solve the following equation:

= (answer: x=1)

1. Method for reducing logarithms to the same base.(slide number 12)

Example:

Solve the log equation 16 x+ log 4 x+ log 2 x=7

ODZ: x>0

¼ log 2 x+½ log 2 x+ log 2 x=7

7/4 log 2 x=7

log 2 x=4

х=16 – belongs to ODZ.

Answer: x=16.

Solve the following equation with the class:

3 (answer: x=5/3)

1. Equations solved by applying the properties of the logarithm.(slide number 13)

Example:

Solve the log equation 2 (x +1) - log 2 (x -2) = 2.

ODZ:

x+1>0;

x-2>0. x>1.

We use the formula for transforming the difference of logarithms of the logarithm of the quotient, we get log 2 = 2, whence follows= 4.

Solving the last equation, we find x \u003d 3, 3\u003e 1 - right

Answer: x = 3.

Solve the following equations with the class:

a) log 5 (x + 1) + log 5 (x +5) = 1 (answer: x=0).

b) log 9 (37-12x) log 7-2x 3 = 1,

37-12x >0, x

7-2x >0, x

7-2x≠ 1; x≠ 3; x≠ 3;

Log 9 (37-12x) / log 3 (7-2x) = 1,

½ log 3 (37-12x) = log 3 (7-2x),

Log 3 (37-12x) = log 3 (7-2x) 2,

37-12x \u003d 49 -28x + 4x 2,

4x 2 -16x +12 \u003d 0,

X 2 -4x +3 \u003d 0, D \u003d 19, x 1 \u003d 1, x 2 =3, 3 is an extraneous root.

Answer: x=1 is the root of the equation.

C) lg (x 2 -6x + 9) - 2lg (x - 7) = lg9.

(x 2 -6x + 9) > 0, x ≠ 3,

X-7 >0; x>7; x>7.

Lg ((x-3)/(x-7)) 2 = lg9

((x-3)/(x-7)) 2 = 9,

(x-3) / (x-7) \u003d 3, (x-3) / (x-7) \u003d - 3,

x-3 \u003d 3x -21, x -3 \u003d - 3x +21,

x=9. x=6 - extraneous root.

The check shows the 9 root of the equation.

Answer: 9

1. Equations solved by introducing a new variable.(slide number 14)

Example:

Solve lg equation 2 x - 6lgx + 5 \u003d 0.

ODZ: x>0.

Let lgx = p, then p 2 -6p+5=0.

p 1 =1, p 2 =5.

Back to replacement:

lgх = 1, lgх =5

x=10, 10>0 – true x=100000, 100000>0 – true

Answer: 10, 100000

Solve the following equation with the class:

Log 6 2 x + log 6 x +14 \u003d (√16 - x 2) 2 + x 2,

16 - x 2 ≥0; - 4≤ x ≤ 4;

X>0, x>0, O.D.Z. [ 0.4).

Log 6 2 x + log 6 x +14 \u003d 16 - x 2 + x 2,

Log 6 2 x + log 6 x -2 = 0

Replace log 6 x = t

T 2 + t -2 \u003d 0; D=9; t 1 \u003d 1, t 2 \u003d -2.

Log 6 x = 1, x = 6 is an extraneous root.

Log 6 x=-2, x=1/36, check shows 1/36 is the root.

Answer: 1/36.

1. Equations Solved by Factoring.(slide number 15)

Example:

Solve the log equation 4 (2x-1) ∙ log 4 x \u003d 2 log 4 (2x-1)

ODZ:

2x-1>0;

X>0. x>½.

log 4 (2x-1)∙ log 4 x - 2 log 4 (2x-1)=0

log 4 (2x-1)∙(log 4 x-2)=0

log 4 (2x-1)=0 or log 4 x-2=0

2x-1=1 log 4 x = 2

x=1 x=16

1;16 - belong to ODZ

Answer: 1;16

Solve the following equation with the class:

log 3 x ∙log 3 (3x-2)= log 3 (3x-2) (answer: x=1)

1. The method of taking the logarithm of both parts of the equation.(slide number 16)

Example:

Solve Equations

Take the logarithm of both sides of the equation in base 3.

We get log 3 = log 3 (3x)

we get: log 3 x 2 log 3 x \u003d log 3 (3x),

2log 3 x log 3 x = log 3 3+ log 3 x,

2 log 3 2 x \u003d log 3 x +1,

2 log 3 2 x - log 3 x -1=0,

replace log 3 x = p, x > 0

2 p 2 + p -2 \u003d 0; D=9; p 1 \u003d 1, p 2 \u003d -1/2

Log 3 x = 1, x=3,

log 3 x \u003d -1 / 2, x \u003d 1 / √3.

Answer: 3; 1/√3

Solve the following equation with the class:

Log 2 x - 1

x \u003d 64 (answer: x \u003d 8; x \u003d 1/4)

1. Functional - graphical method.(slide number 17)

Example:

Solve equations: log 3 x = 12 x.

Since the function y = log 3 x is increasing, and the function y \u003d 12 x is decreasing on (0; + ∞), then the given equation on this interval has one root.

Let's build graphs of two functions in one coordinate system: y = log 3 x and y = 12 x.

At x=10, the given equation turns into the correct numerical equality 1=1. The answer is x=10.

Solve the following equation with the class:

1-√x \u003d ln x (answer: x \u003d 1).

1. Summing up, reflection (hand out circles on which the guys mark their mood with a picture).(slide number 18,19)

Determine the method for solving the equation:

1. Homework: 340(1), 393(1), 395(1.3), 1357(1.2), 337(1), 338(1), 339(1)

Literature

1. Ryazanovsky, A.R. Maths. Grades 5 - 11: Additional materials for the lesson of mathematics / A.R. Ryazanovsky, E.A. Zaitsev. - 2nd ed., stereotype. - M .: Bustard, 2002
2. Maths. Supplement to the newspaper "First of September". 1997. No. 1, 10, 46, 48; 1998. No. 8, 16, 17, 20, 21, 47.
3. Skorkina, N.M. Non-standard forms of extracurricular work. For middle and high school / N.M. Skorkin. - Volgograd: Teacher, 2004
4. Ziv, B.G., Goldich, V.A. Didactic materials on algebra and principles of analysis for grade 10./B.G.Ziv, V.A.Goldich. - 3rd ed., corrected. - St. Petersburg: "CheRo-on-Neva", 2004
5. Algebra and the Beginnings of Analysis: Mathematics for Technical Schools / ed. G.N. Yakovleva.-M.: Nauka, 1987

Preview:

To use the preview of presentations, create a Google account (account) and sign in: https://accounts.google.com

Slides captions:

Methods for solving logarithmic equations Mathematics teacher: Plotnikova T.V. MBOU "Secondary School No. 1 of Suzdal"

Definition The logarithm of a positive number b to the base a, where a>0, a≠1, is such an exponent c, to which you need to raise ato get b.

Properties of logarithms log a 1 = 0 log a a = 1 log a (x y)= log a x + log a y 3

Base Transfer Formulas 4

Calculate: 5

Compare 6

7 Determine the sign of the number:

Basic methods for solving logarithmic equations

1. Using the definition of the logarithm l og 2 128= x log x 27= 3 Solve the following equations: a) log 7 (3x-1)=2 b) log 2 (7-8x)=2 9

2. Method of potentiation Let's solve the following equation: lg (x 2 -2) = lg x 10 2

11 3. Equations Solved by Applying the Basic Logarithmic Identity Let's solve the following equation: 1

12 4 . Method for reducing logarithms to the same base log 16 x + log 4 x + log 2 x = 7 Solve the following equation:

13 5. Equations solved by applying the properties of the logarithm log 2 (x +1) - log 2 (x -2) \u003d 2 We solve the following equations: a) l og 5 (x +1) + log 5 (x +5) \u003d 1 b) log 9 (37-12x) log 7-2x 3 \u003d 1 c) lg (x 2 -6x + 9) - 2lg (x - 7) \u003d lg9 0 1 9

6. Equations solved by introducing a new variable l g 2 x - 6lgx +5 = 0 We solve the following equations: log 6 2 x + log 6 x +14 = (√16 - x 2) 2 + x 2 14

15 7. Equations Solved by Factoring log 4 (2x-1)∙ log 4 x =2 log 4 (2x-1) Solve the following equations: log 3 x ∙ log 3 (3x-2)= log 3 ( 3x-2) 1

8. Logarithm method Let's solve the following equation: 16

9. Functionally - graphical method log 3 x = 12-x Let's solve the following equation: 17 1

Determine the method for solving the equation: Equation: Solving method for determining the logarithm transition to another base factorization potentiation introduction of a new variable transition to another base use of the properties of the logarithm logarithm graphic 18

Yes! And who came up with these logarithmic equations! I can do everything!!! Need a couple more examples? Reflection 19

Algebra Grade 11

Topic: "Methods for solving logarithmic equations"

Lesson Objectives:

educational: the formation of knowledge about different ways of solving logarithmic equations, the ability to apply them in each specific situation and choose any method for solving;

developing: development of skills to observe, compare, apply knowledge in a new situation, identify patterns, generalize; formation of skills of mutual control and self-control;

educational: education of a responsible attitude to educational work, careful perception of the material in the lesson, accuracy of record keeping.

Lesson type : a lesson of familiarization with new material.

"The invention of logarithms, by shortening the work of the astronomer, has lengthened his life."
French mathematician and astronomer P.S. Laplace

During the classes

I. Setting the goal of the lesson

The studied definition of the logarithm, the properties of logarithms and the logarithmic function will allow us to solve logarithmic equations. All logarithmic equations, no matter how complex they are, are solved using the same algorithms. We will consider these algorithms today in the lesson. There are few of them. If you master them, then any equation with logarithms will be feasible for each of you.

Write in your notebook the topic of the lesson: "Methods for solving logarithmic equations." I invite everyone to cooperation.

II. Updating of basic knowledge

Let's get ready to study the topic of the lesson. You solve each task and write down the answer, you can not write the condition. Work in pairs.

1) For what values ​​of x does the function make sense:

a)

b)

in)

e)

(Answers are checked for each slide and errors are sorted out)

2) Do the function graphs match?

a) y = x and

b)and

3) Rewrite the equalities as logarithmic equalities:

4) Write the numbers as logarithms with base 2:

4 =

2 =

0,5 =

1 =

5) Calculate :

6) Try to restore or complete the missing elements in these equalities.

III. Introduction to new material

The statement is shown on the screen:

"The equation is the golden key that unlocks all mathematical sesame."
Modern Polish mathematician S. Koval

Try to formulate the definition of a logarithmic equation. (An equation containing an unknown under the sign of the logarithm ).

Considerthe simplest logarithmic equation: log a x = b (where a>0, a ≠ 1). Since the logarithmic function increases (or decreases) on the set of positive numbers and takes all real values, it follows from the root theorem that for any b, this equation has, and moreover, only one solution, and a positive one.

Remember the definition of a logarithm. (The logarithm of the number x to the base a is the exponent to which the base a must be raised to get the number x ). It immediately follows from the definition of the logarithm thata in is such a solution.

Write down the title:Methods for solving logarithmic equations

1. By definition of the logarithm .

This is how the simplest equations of the form.

ConsiderNo. 514(a ): Solve the equation

How do you propose to solve it? (By definition of the logarithm )

Decision . 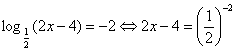, Hence 2x - 4 = 4; x = 4.

Answer: 4.

In this task, 2x - 4 > 0, since> 0, so no extraneous roots can appear, andverification is not necessary . The condition 2x - 4 > 0 in this task is not necessary to write out.

2. Potentiation (transition from the logarithm of the given expression to this expression itself).

ConsiderNo. 519(g): log 5 ( x 2 +8)- log 5 ( x+1)=3 log 5 2

What feature did you notice?(The bases are the same and the logarithms of the two expressions are equal) . What can be done?(potentiate).

In this case, it should be taken into account that any solution is contained among all x for which the logarithm expressions are positive.

Decision: ODZ:

X 2 +8>0 extra inequality

log 5 ( x 2 +8) = log 5 2 3 + log 5 ( x+1)

log 5 ( x 2 +8)= log 5 (8 x+8)

Potentiate the original equation

x 2 +8= 8 x+8

we get the equationx 2 +8= 8 x+8

Let's solve it:x 2 -8 x=0

x=0, x=8

Answer: 0; eight

In generaltransition to an equivalent system :

The equation

(The system contains a redundant condition - one of the inequalities can be ignored).

Question to the class : Which of these three solutions did you like the most? (Discussion of methods).

You have the right to decide in any way.

3. Introduction of a new variable .

ConsiderNo. 520(g) . .

What did you notice? (This is a quadratic equation for log3x) Your suggestions? (Introduce new variable)

Decision . ODZ: x > 0.

Let be, then the equation will take the form:. Discriminant D > 0. Roots by Vieta's theorem:.

Back to replacement:or.

Solving the simplest logarithmic equations, we get:

; .

Answer : 27;

4. Logarithm of both sides of the equation.

Solve the equation:.

Decision : ODZ: x>0, we take the logarithm of both sides of the equation in base 10:

. Apply the property of the logarithm of the degree:

(lgx + 3) lgx =

(lgx + 3) lgx = 4

Let lgx = y, then (y + 3)y = 4

, (D > 0) the roots according to the Vieta theorem: y1 = -4 and y2 = 1.

Let's go back to the replacement, we get: lgx = -4,; logx = 1,. . It is as follows: if one of the functions y = f(x) increases and the other y = g(x) decreases on the interval X, then the equation f(x)=g(x) has at most one root on the interval X .

If there is a root, then it can be guessed. .

Answer : 2

“The correct application of methods can be learned,
only by applying them to various examples.
Danish historian of mathematics G. G. Zeiten

I V. Homework

P. 39 consider example 3, solve No. 514 (b), No. 529 (b), No. 520 (b), No. 523 (b)

V. Summing up the lesson

What methods for solving logarithmic equations did we consider in the lesson?

In the next lessons, we will look at more complex equations. To solve them, the studied methods are useful.

Showing the last slide:

“What is more than anything in the world?
Space.
What is the wisest?
Time.
What is the most enjoyable?
Achieve what you want."
Thales

I want everyone to achieve what they want. Thank you for your cooperation and understanding.